Algebraic Equations Worksheets 7th Grade

Algebraic equations worksheets are a valuable resource for 7th grade students who are learning the fundamentals of algebra. These worksheets provide a structured and organized way for students to practice solving equations, giving them the opportunity to reinforce their understanding of this important mathematical concept. By working through various equations, students can develop their problem-solving skills and become more confident in tackling algebraic problems in their coursework.

  7th Grade Math Algebra Equations Worksheets

---

  Solving Equations Worksheets 7th Grade Math

---

  Math Expressions Worksheets 7th Grade

---

  8th Grade Algebraic Equations Worksheets

---

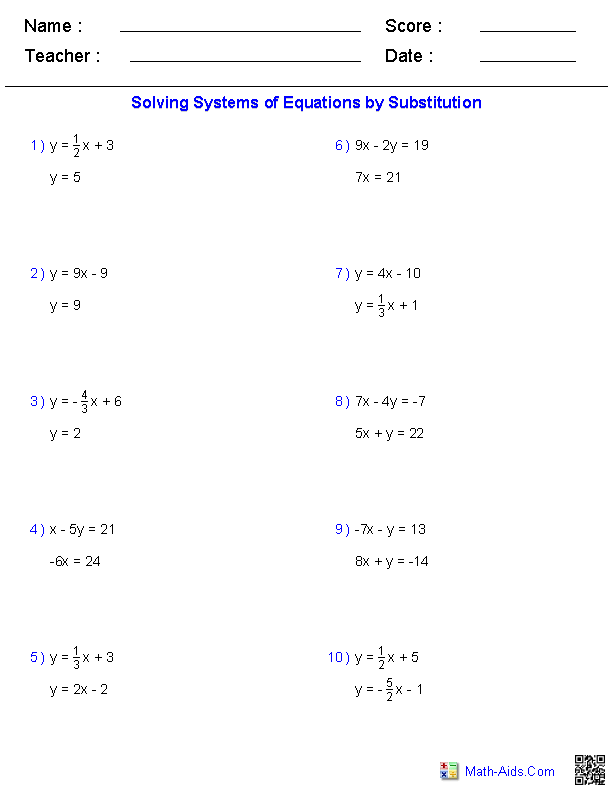
  Two-Step Equations Worksheets 8th Grade Math

---

  Pre-Algebra 7th Grade Math Worksheets

---

  7th Grade Math Worksheets

---

  Algebra 1 Worksheets

---

  7th Grade Math Worksheets Algebra

---

  7th Grade Math Worksheets Algebra

---

  7th Grade Pre-Algebra Worksheets

---

  5th Grade Algebra Variables Worksheets

---

What is an algebraic equation?

An algebraic equation is a mathematical expression that uses numbers, variables, and mathematical operations to show the equality of two expressions. It typically involves solving for an unknown variable by manipulating the equation using the rules of algebra.

What is the difference between an expression and an equation?

An expression is a mathematical phrase that contains numbers, variables, and operations but does not have an equal sign, while an equation is a mathematical statement that shows equality between two expressions with an equal sign. In other words, an expression represents a quantity or value, whereas an equation shows a relationship between two quantities.

How do you solve a simple algebraic equation with one variable?

To solve a simple algebraic equation with one variable, isolate the variable by performing the same operation on both sides of the equation. Start by simplifying the equation using the order of operations, then combine like terms. Next, move constants to one side of the equation and variables to the other side using addition or subtraction. Finally, solve for the variable by performing the necessary operations to isolate it and find the solution. Remember to perform the same operation to both sides to maintain the equality of the equation.

What are the steps for solving a multi-step equation?

To solve a multi-step equation, start by simplifying both sides of the equation using the order of operations (PEMDAS). Combine like terms and simplify each side as much as possible. Then isolate the variable by performing inverse operations in the opposite order they appear in the equation. These operations can include addition, subtraction, multiplication, and division. Finally, check your solution by substituting it back into the original equation to ensure that it satisfies the equation.

How do you solve an equation with fractions or decimals?

To solve an equation with fractions or decimals, you may start by clearing out any fractions by multiplying both sides of the equation by the least common multiple (LCM) of the denominators. This will eliminate the fractions and allow you to work with whole numbers. Then, you can proceed to solve the equation as you would with any other type of equation, following the order of operations and simplifying steps until you isolate the variable on one side of the equation. Finally, make sure to check your solution by substituting it back into the original equation to verify its accuracy.

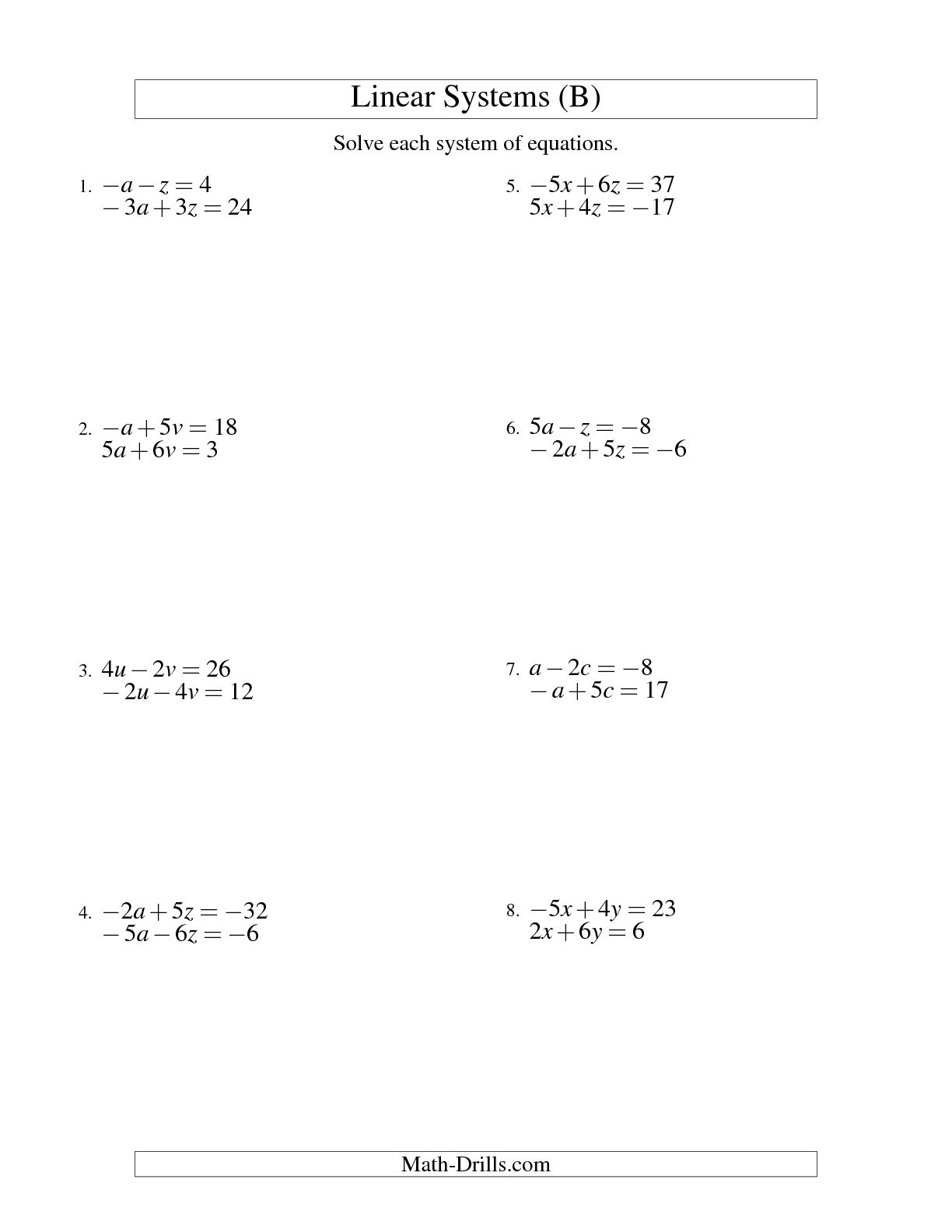
What is the process for solving an equation with variables on both sides?

To solve an equation with variables on both sides, the first step is to simplify both sides by combining like terms. Next, move all variable terms to one side of the equation and all constant terms to the other side by performing inverse operations. Divide or multiply if necessary to isolate the variable on one side. Finally, solve for the variable by computing the value that satisfies the equation. Always remember to perform the same operation on both sides to maintain the equality of the equation.

How do you solve equations with variables on one side and constants on the other?

To solve equations with variables on one side and constants on the other, you need to isolate the variable by performing inverse operations. This involves undoing addition and subtraction by performing the opposite operation, then undoing multiplication and division by performing the inverse operation. By following these steps, you can find the value of the variable that satisfies the equation.

What are literal equations and how are they solved?

Literal equations are equations that involve multiple variables. To solve a literal equation, you manipulate the equation algebraically to isolate the variable you want to solve for. This typically involves using inverse operations such as addition, subtraction, multiplication, and division to isolate the variable on one side of the equation. By performing the same operations on both sides of the equation, you can determine the value of the variable.

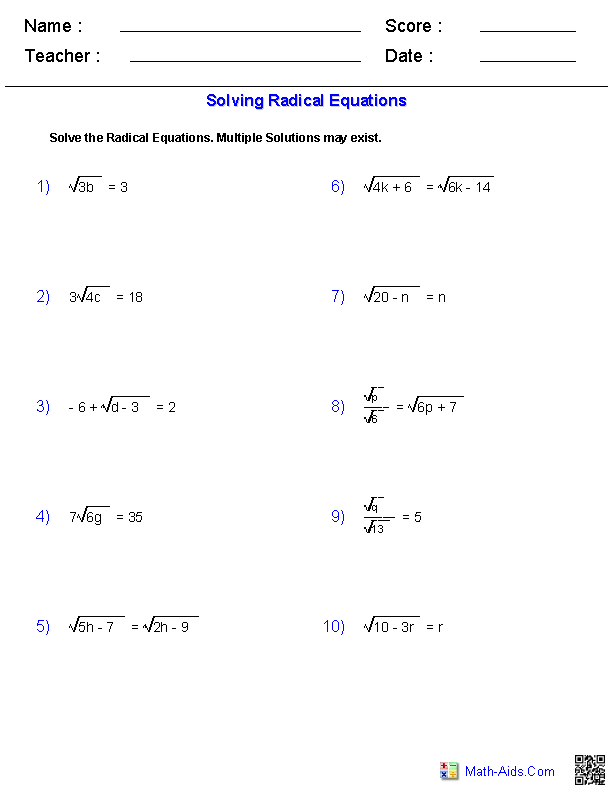
How do you solve equations involving exponents or radicals?

To solve equations involving exponents or radicals, you typically isolate the term with the exponent or radical and then apply inverse operations to both sides of the equation. For equations with exponents, you can raise both sides to the reciprocal power to eliminate the exponent. For equations with radicals, you raise both sides to a power that will eliminate the radical. Remember to check your solutions, as extraneous solutions may occur when working with radicals. Practice and familiarity with properties of exponents and radicals will help in confidently solving equations involving these mathematical concepts.

What is the importance of checking your solution after solving an equation?

Checking your solution after solving an equation is important to ensure that you have not made any errors during the calculation process. It helps verify that the solution you have found is accurate and meets the requirements of the original problem. By double-checking your work, you can catch any mistakes and make corrections if needed, increasing the reliability and credibility of your final answer.