Algebra Coordinate Plane Worksheets

Algebra Coordinate Plane Worksheets are designed to assist students in mastering the fundamental concepts of graphing equations on a coordinate plane. These worksheets provide a comprehensive range of practice problems and exercises that focus on key topics such as plotting points, identifying quadrants, graphing lines, and solving equations. With a precise emphasis on building a solid foundation in algebra, these worksheets are the perfect resource for students who wish to enhance their understanding of coordinate planes and develop their graphing skills.

  Four Coordinate Graph Paper

---

  Coordinate Plane Ideas

---

  Printable Coordinate Graph Paper

---

  Graphing Coordinate Plane Worksheet

---

  Coordinate Graph Paper with Numbers

---

  Coordinate Graph Mystery

---

  Coordinate Grid Graph Paper Printable

---

  Coordinate Plane Grid Paper

---

  Geometry Dilations Worksheet

---

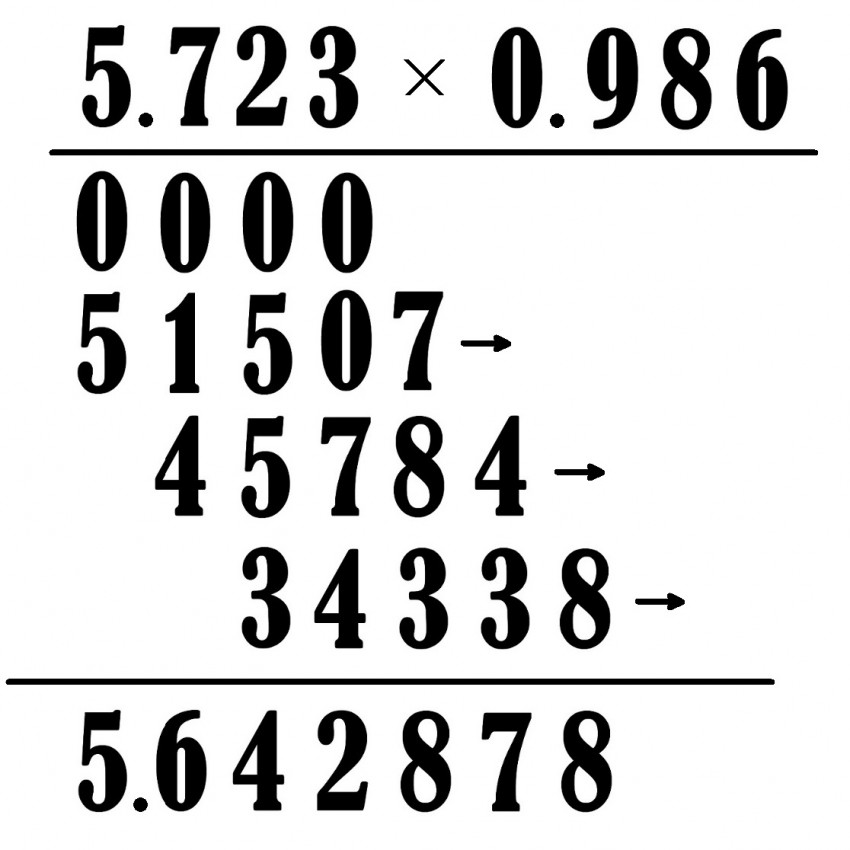
  Multiplication of Decimals Worksheet

---

  Math Graph Paper Template

---

What is the coordinate plane?

The coordinate plane is a two-dimensional plane formed by two perpendicular number lines, called the x-axis and y-axis. It is used to locate points using ordered pairs of numbers, with the x-coordinate representing the horizontal position and the y-coordinate representing the vertical position. Together, these coordinates allow for precise positioning and measuring distances between points in geometry and algebraic equations.

How do you locate a point on the coordinate plane?

To locate a point on the coordinate plane, you use the x-coordinate (horizontal position) and the y-coordinate (vertical position) of the point. Plot the point where the x and y coordinates intersect on the graph, with the x-coordinate determining the left-right position and the y-coordinate determining the up-down position. The point's location is determined by its specific x and y values on the graph.

What are the quadrants of the coordinate plane?

The quadrants of the coordinate plane are four sections into which the plane is divided, based on the signs of the x and y coordinates. The quadrants are named as follows: the first quadrant where both x and y values are positive, the second quadrant where x is negative and y is positive, the third quadrant where both x and y values are negative, and the fourth quadrant where x is positive and y is negative.

How do you find the distance between two points on the coordinate plane?

To find the distance between two points on the coordinate plane, you can use the distance formula, which is derived from the Pythagorean theorem. The formula is: sqrt((x2 - x1)^2 + (y2 - y1)^2), where (x1, y1) and (x2, y2) represent the coordinates of the two points. Simply substitute the coordinates into the formula and calculate the distance to get the answer.

What is the midpoint formula and how is it used on the coordinate plane?

The midpoint formula is used to find the midpoint between two points on a coordinate plane. The formula is ( (x1 + x2) / 2, (y1 + y2) / 2 ), where (x1, y1) and (x2, y2) are the coordinates of the two points. This formula calculates the average of the x-coordinates and the average of the y-coordinates to determine the point that is equidistant from the two given points. It is commonly used in geometry and algebra to find the center or middle point between two locations.

How do you graph linear equations on the coordinate plane?

To graph a linear equation on the coordinate plane, start by rearranging the equation into slope-intercept form (y = mx + b), where m represents the slope and b is the y-intercept. Plot the y-intercept on the y-axis, then use the slope to find additional points by moving along the line based on the rise over run. Finally, connect the points to create the straight line that represents the linear equation on the coordinate plane.

How do you determine the slope of a line on the coordinate plane?

To determine the slope of a line on the coordinate plane, you need to calculate the change in the y-coordinates divided by the change in the x-coordinates of two distinct points on the line. This is represented by the formula: (y2 - y1)/(x2 - x1), where (x1, y1) and (x2, y2) are the coordinates of the two points. The resulting value is the slope of the line, which indicates the steepness or incline of the line.

What is the equation of a horizontal line on the coordinate plane?

The equation of a horizontal line on the coordinate plane is y = c, where c is a constant value representing the y-coordinate of all points on that line. This means that no matter what the x-coordinate is, the y-coordinate will always be equal to c, resulting in a straight line parallel to the x-axis.

How do you find the equation of a vertical line on the coordinate plane?

To find the equation of a vertical line on the coordinate plane, you use the general form x = a, where 'a' is the x-coordinate of any point on the line. This is because all points on a vertical line have the same x-coordinate. Therefore, the equation of a vertical line is simply x = a, where 'a' is a constant.

How can the coordinate plane be used to solve real-world problems involving algebraic equations?

The coordinate plane can be used to solve real-world problems involving algebraic equations by representing variables as points or lines on the graph, allowing us to visualize and interpret the relationships between quantities. By graphing equations on the coordinate plane, we can find points of intersection to solve for unknown values, determine possible solutions, analyze trends, and make predictions based on the data. This visual representation helps in understanding and solving equations in real-world scenarios such as budgeting, planning, or problem-solving.