Algebra 1 Multiplying Polynomials Worksheet
Are you a high school student studying Algebra 1 and looking to practice multiplying polynomials? If so, you're in the right place! In this blog post, we will explore the benefits of using worksheets to reinforce your understanding of this important algebraic concept. By providing a variety of exercises that focus on multiplying polynomials, these worksheets serve as valuable tools for mastery and improvement in this specific area of mathematics.
Table of Images 👆
- Factoring Polynomials Worksheet
- Kuta Software Infinite Algebra 1 Multiplying Polynomials
- Algebra 1 Factoring Problems and Answers
- Algebra 1 Worksheets
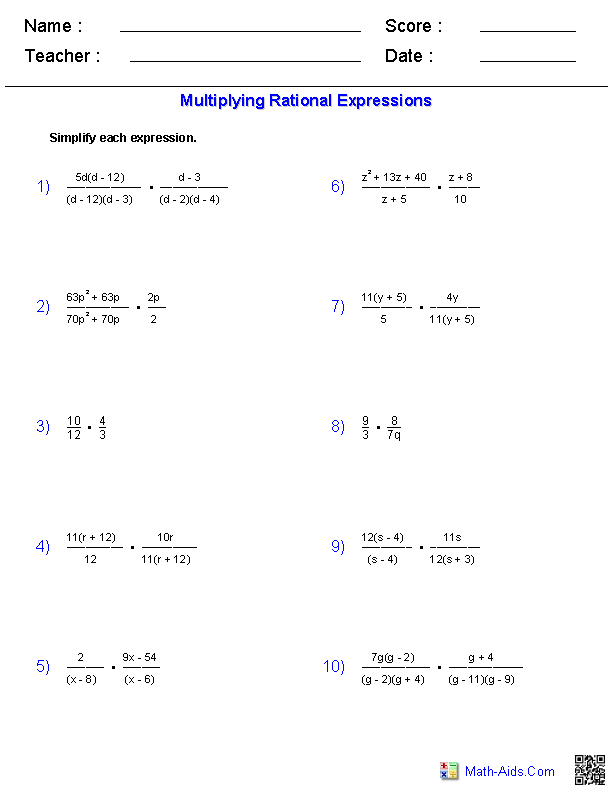
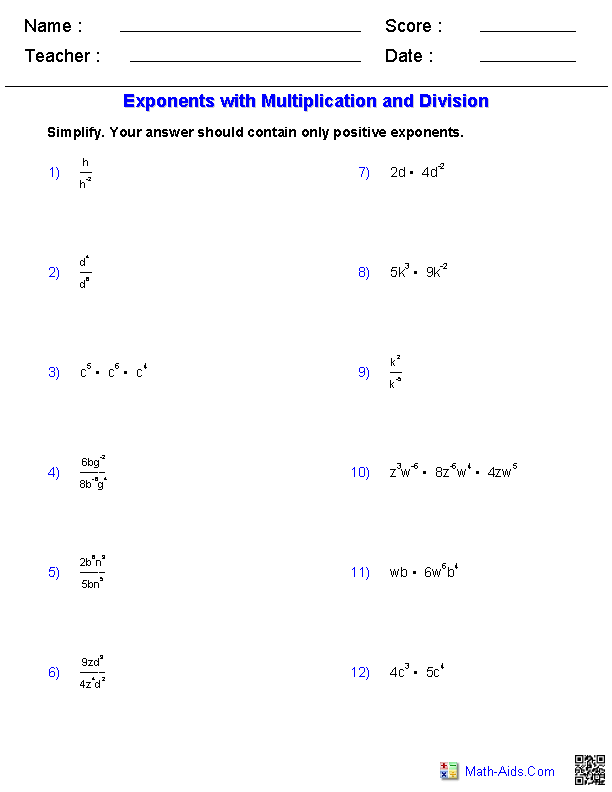
- Multiplication of Exponents and Division Worksheets
- Algebra 2 Factoring Polynomials Worksheet 1
- Algebra Factoring Polynomials Worksheet
- Polynomials and Factoring Practice Worksheet Answers
- Multiplying Polynomials Worksheet with Answers
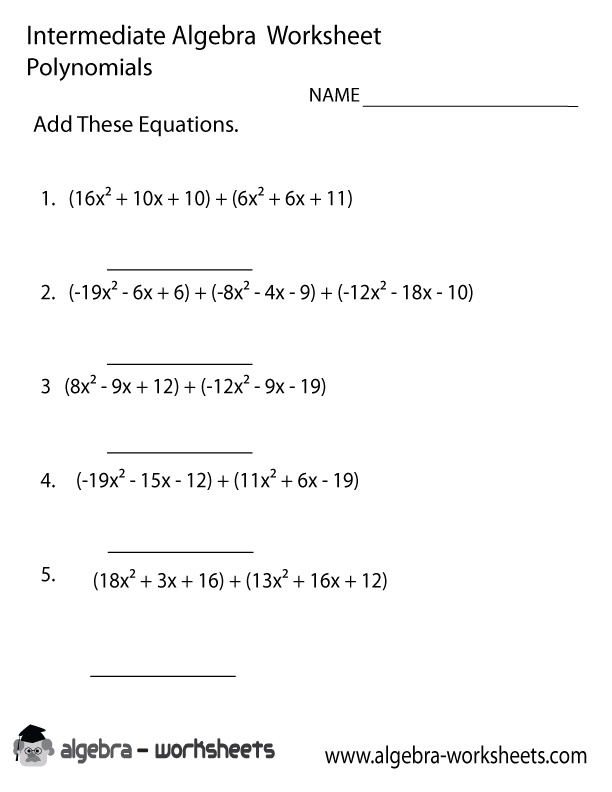
- Algebra Polynomials Worksheets
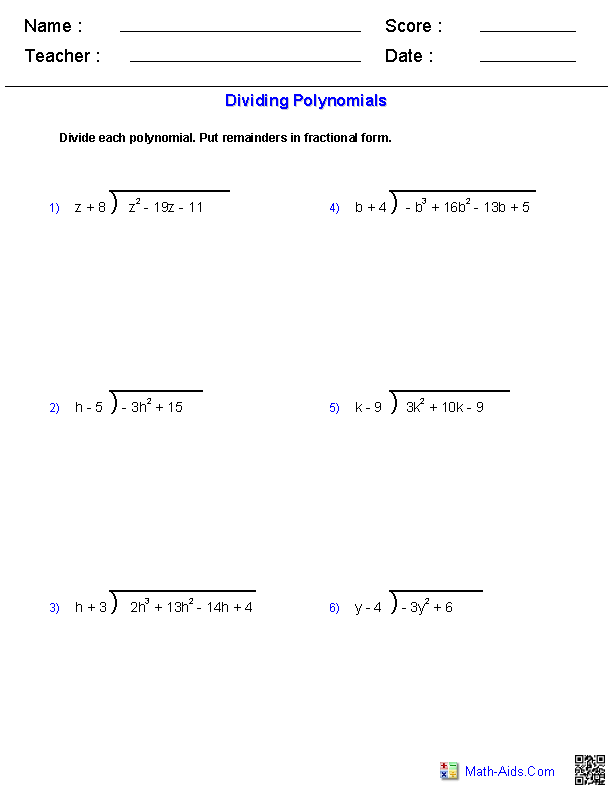
- 6th Grade Long Division Worksheets
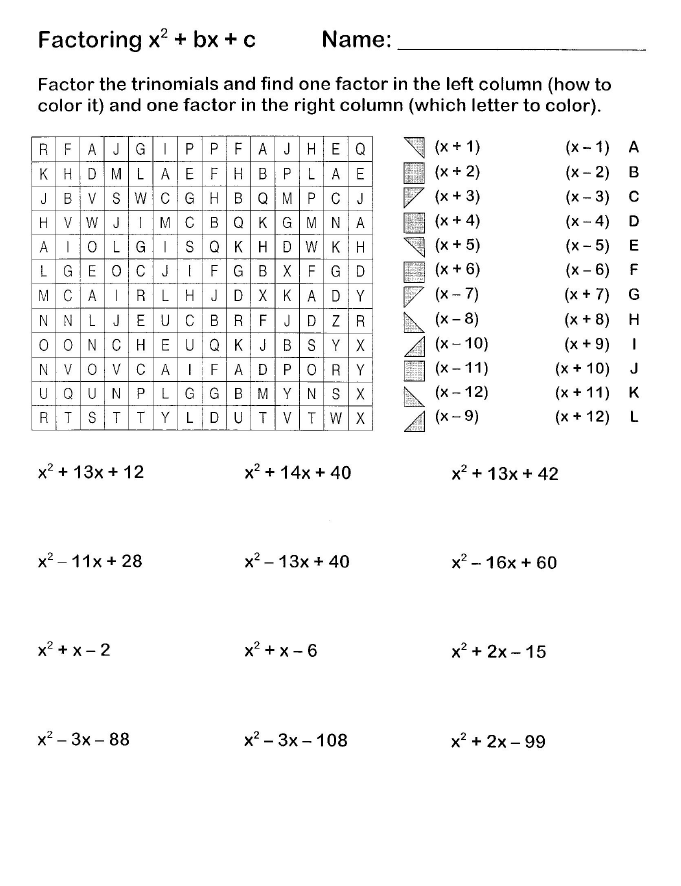
- Factoring Trinomials Worksheet Coloring
- Multiplying and Factoring Polynomials Worksheet
- Factoring Trinomials Practice Worksheet
- Algebra 2 Factoring Review Worksheet Answers
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Art Handouts and Worksheets
7 Elements of Art Worksheets
What is the first step in multiplying two polynomials?
The first step in multiplying two polynomials is to distribute each term of the first polynomial across every term of the second polynomial, ensuring that each term is multiplied by every other term.
How do you determine the degree of the product of two polynomials?
To determine the degree of the product of two polynomials, you simply multiply the degrees of the two polynomials. For example, if you have a polynomial of degree 2 and another polynomial of degree 3, the degree of their product will be 2 + 3 = 5. This is because when you multiply two polynomials, the highest degree term in the resulting polynomial will have a degree equal to the sum of the degrees of the original polynomials.
What is the distributive property and how is it applied in polynomial multiplication?
The distributive property states that for any real numbers a, b, and c, a multiplied by the sum of b and c is equal to the product of a and b added to the product of a and c. In polynomial multiplication, the distributive property is applied by multiplying each term in one polynomial by every term in the other polynomial, and then adding the resulting products together. This process involves distributing each term in one polynomial across the terms of the other polynomial, hence the name "distributive property.
Can you explain the concept of a monomial and how it relates to polynomial multiplication?
A monomial is a single term in algebra consisting of a coefficient and one or more variables raised to non-negative integer exponents. When multiplying polynomials, you essentially distribute each term in one polynomial by each term in the other polynomial, which includes monomials. This means that when you multiply two polynomials together, you are essentially combining all the individual monomial terms by following the rules of exponents and coefficients to simplify the expression. Each term in the resulting polynomial will be a combination of the terms from the original polynomials, including the monomials.
What is the significance of the zero exponent when multiplying polynomials?
The zero exponent signifies that when a polynomial is raised to the power of zero, the result is always 1. This is a fundamental property in mathematics that allows for simplification of polynomial expressions and helps in performing various calculations involving polynomials efficiently.
How do you handle multiplication of polynomials with multiple terms and exponents?
To handle multiplication of polynomials with multiple terms and exponents, you need to use the distributive property. Multiply each term in the first polynomial by every term in the second polynomial, then combine like terms by adding or subtracting their coefficients. Keep track of the exponents of each variable and apply the rules of exponents when multiplying like terms. Finally, simplify the resulting expression by combining like terms to get the final product of the two polynomials.
What is the purpose of using FOIL when multiplying binomials?
The purpose of using FOIL (First, Outer, Inner, Last) when multiplying binomials is to systematically distribute each term of the first binomial to every term of the second binomial, ensuring that no terms are overlooked and all products are accounted for in the final result. This method helps organize the multiplication process and makes it easier to keep track of each multiplication step, leading to an accurate and complete expansion of the binomials.
Can you elaborate on the concept of factoring and its role in polynomial multiplication?
Factoring in polynomial multiplication involves breaking down a polynomial into smaller, simpler polynomials that can be multiplied together. This simplifies the process of multiplying polynomials by reducing the number of operations required. By factoring a polynomial, we can identify common terms and simplify the expression, making it easier to solve algebraic problems and equations efficiently. Moreover, factoring also helps in understanding the relationships and patterns within polynomial expressions, making it a crucial technique in algebraic manipulations and problem-solving strategies.
What are some common mistakes to avoid when multiplying polynomials?
Some common mistakes to avoid when multiplying polynomials include incorrectly distributing terms, forgetting to multiply terms in each set of parentheses, mixing up the order of terms, not combining like terms properly, and incorrectly applying the distributive property. It is important to carefully follow the steps of the multiplication process and double-check your work to ensure accuracy.
How can you use polynomial multiplication to solve real-life problems?
Polynomial multiplication can be used to solve real-life problems such as calculating area and volume of geometric shapes, determining profit margins in business, analyzing trends in data sets, and predicting future values in statistics. By representing these scenarios as polynomials and multiplying them together, we can obtain more accurate and detailed solutions to various practical problems.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.








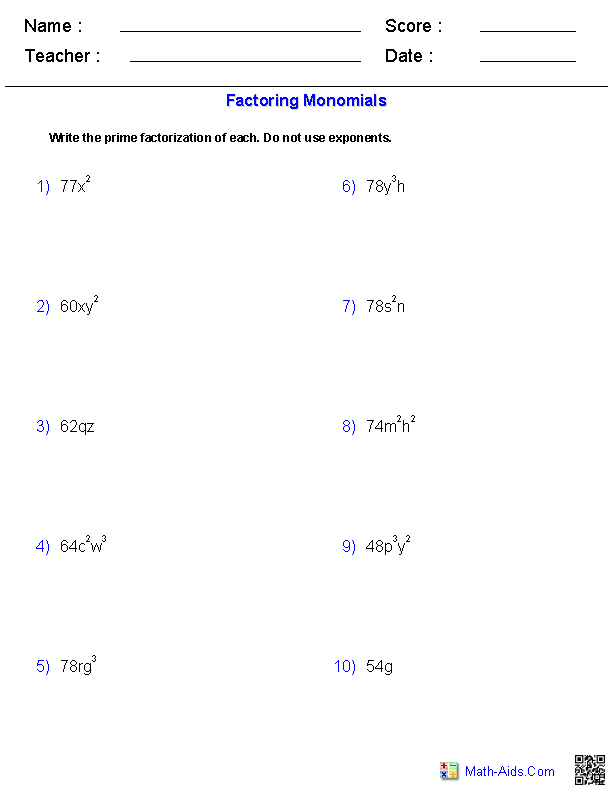


















Comments