Absolute Value Graphs Worksheets

Absolute value graphs worksheets provide students with valuable practice in understanding and interpreting the concept of absolute value functions. These worksheets are designed to help students develop their skills in graphing and analyzing the behavior of absolute value equations. Whether you are a teacher looking for additional resources for your students or a student who wants to strengthen their understanding of absolute value graphs, these worksheets are a helpful tool that provides essential practice.

  Fifth Grade Math Worksheets

---

  9th Grade Algebra Equations Worksheets

---

  Adding Negative Numbers Worksheet

---

  Place Value Worksheets 2nd Grade

---

  Frame Graphic Organizer Printable

---

  Printable Christmas Graph Worksheets

---

  Domain and Range Worksheets

---

  8th Grade Math Worksheets Algebra

---

  Graphing Linear Equations Algebra 1

---

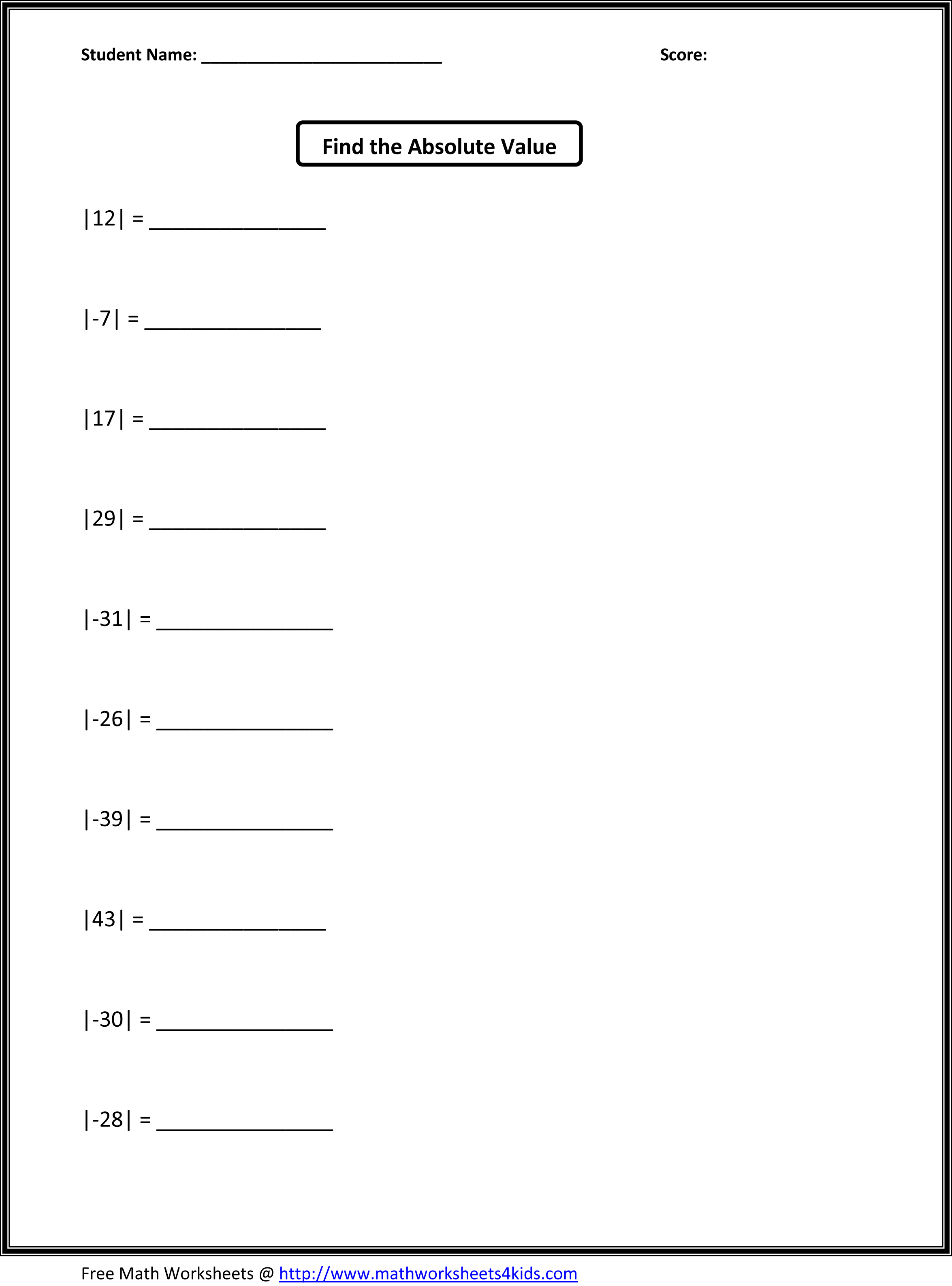
What does the absolute value of a number represent?

The absolute value of a number represents the distance of that number from zero on the number line. It gives the magnitude or size of a number without considering its sign, always resulting in a non-negative value.

How does the graph of y = |x| differ from the graph of y = x?

The graph of y = |x| is the absolute value of x, which means that it reflects negative values of x across the y-axis to make them positive. This results in a V-shaped graph with its vertex at the origin. On the other hand, the graph of y = x is a straight line passing through the origin with a slope of 1. So, the main difference is that the graph of y = |x| only shows positive values of x, reflected across the y-axis, while the graph of y = x shows all values of x in a straight line.

How do you graph y = |x|?

To graph y = |x|, you would plot the absolute value function as a V-shaped graph. The graph increases to the right and left of the y-axis, mirroring the positive values of x and reflecting negative values of x. The graph passes through the origin (0,0) with a slope of 1. Thus, the resulting graph is a symmetric V-shape with its vertex at the origin.

What are the key characteristics of the absolute value graph?

The key characteristics of the absolute value graph include a "V" shape, symmetry across the y-axis, passing through the origin, and always positive values. The graph is also linear, with a slope of 1 for the positive side and -1 for the negative side, showing a sharp turn at the vertex point.

What is the shape of an absolute value graph?

The graph of an absolute value function typically forms a "V" shape. It is symmetrical around the y-axis and has a vertex at the point where the function's argument is zero. The graph extends upward and downward from the vertex along the y-axis, while the lines on either side of the vertex have a slope of 1.

How does the coefficient before the absolute value affect the graph?

The coefficient before the absolute value in an absolute value function affects the vertical stretch or compression of the graph. If the coefficient is greater than 1, it will stretch the graph vertically. If it is between 0 and 1, it will compress the graph vertically. Conversely, if it is negative, it will flip the graph vertically. The larger the coefficient, the more stretched or compressed the graph will be.

How can you determine the x-intercepts of an absolute value graph?

To determine the x-intercepts of an absolute value graph, set the absolute value expression inside the graph equal to zero and solve for x. This means finding the values of x where the absolute value of x is equal to zero. Since the absolute value of any number is always positive, the only time the absolute value will be zero is when the number inside the absolute value is zero. So, the x-intercepts of an absolute value graph occur at the values of x that make the expression inside the absolute value equal to zero.

How do you find the vertex of an absolute value function?

The vertex of an absolute value function in the form y = |ax + b| is found by setting the expression inside the absolute value bars (ax + b) equal to zero to find the x-coordinate of the vertex. Then, substitute this x-value back into the absolute value function to find the corresponding y-coordinate of the vertex.

How do you calculate the slope of an absolute value graph?

To calculate the slope of an absolute value graph, you need to consider the two possible slopes that occur on either side of the vertex of the V-shaped graph. The slope to the left of the vertex is typically -1, and the slope to the right of the vertex is typically +1. However, it is important to note that the slope can differ based on the specific equation of the absolute value graph.

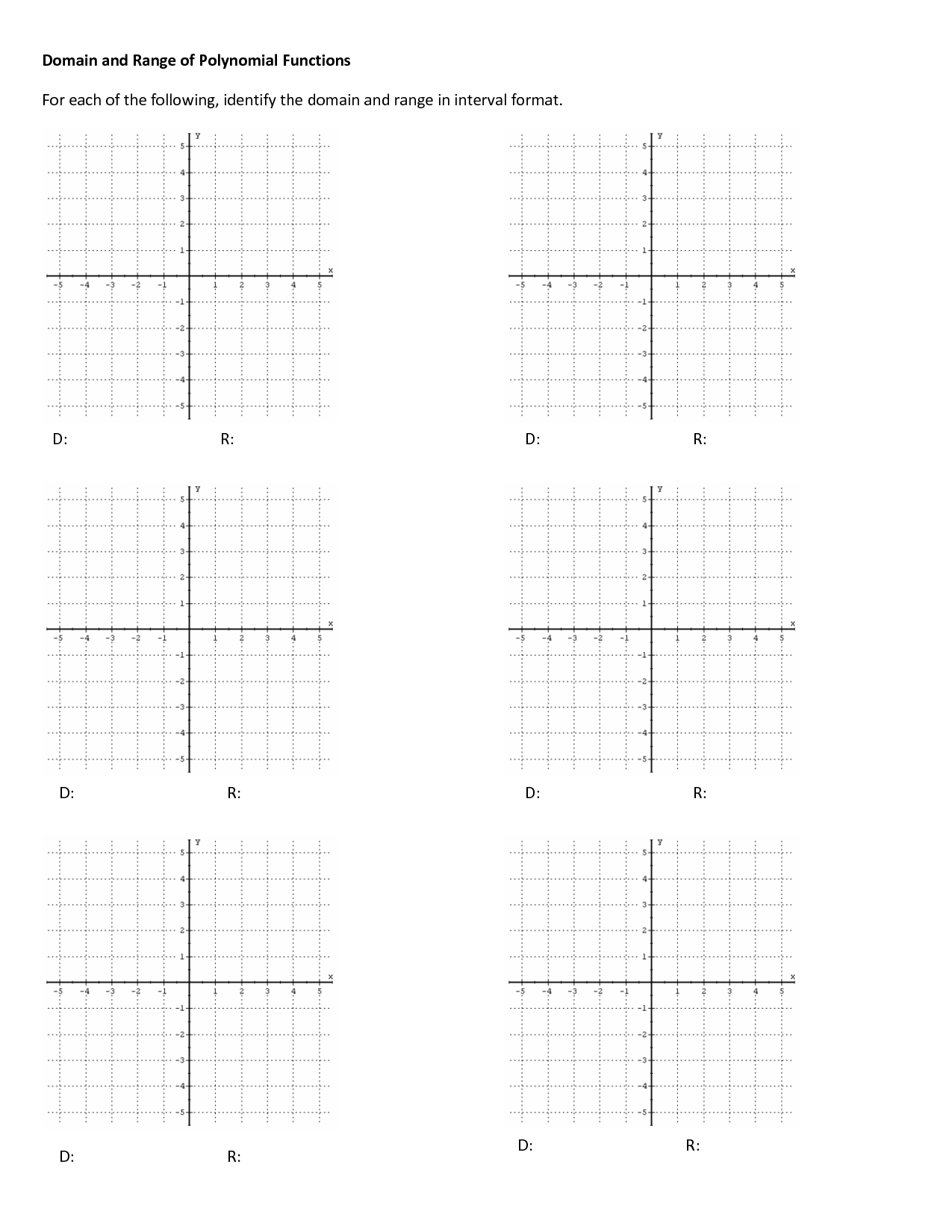
How do you describe the domain and range of an absolute value graph?

The domain of an absolute value graph is all real numbers, as the function can accept any input. However, the range of an absolute value graph is limited to non-negative real numbers, starting from zero and extending infinitely upwards on the y-axis. This is because the absolute value function always yields a non-negative result.