6 Grade Algebra Worksheets
If you're searching for comprehensive and engaging algebra worksheets tailored specifically for 6th graders, you're in the right place. These worksheets focus on introducing key algebraic concepts and building a strong foundation in algebra for students at this grade level.
Table of Images 👆
- 8th Grade Math Worksheets Algebra
- 6th Grade Math Worksheets Angles
- Basic Algebraic Expression Worksheets
- 3 Grade Math Worksheets
- Algebra 1 Practice Worksheets
- 6th-Grade Integers Worksheets
- 7th Grade Math Worksheets Algebra
- 7th Grade Math Worksheets
- Patterns and Algebra Worksheets
- Order of Operations Worksheets 6th Grade
- 6th Grade Math Worksheets with Answer Key
- Multi-Step Multiplication Word Problems
- Math Reference Sheet Grade 7
- 8th Grade Math Worksheets Geometry
- 8th Grade Math Worksheets Geometry
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Healthy Eating Plate Printable Worksheet
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Rosa Parks Worksheet Grade 1
What is the distributive property?
The distributive property states that for any numbers a, b, and c, the product of a and the sum of b and c is equal to the sum of the products of a and b and a and c. In other words, a(b + c) = ab + ac. This property is widely used in algebraic equations to simplify expressions and solve equations.
How do you simplify algebraic expressions?
To simplify algebraic expressions, you should combine like terms by adding or subtracting coefficients of variables with the same type and exponent. You can also apply the distributive property by factoring out common factors and then combine like terms. Remember to follow the order of operations (PEMDAS) and be careful with negative signs. Finally, simplify any fractions or powers by dividing or multiplying accordingly.
How do you solve one-step equations?
To solve one-step equations, isolate the variable by performing the inverse operation on both sides of the equation. For example, if the equation is 2x + 4 = 10, to isolate x, subtract 4 from both sides, yielding 2x = 6. Then, divide both sides by 2 to find the value of x, giving x = 3. Remember to always perform the same operation on both sides to maintain the equality of the equation.
What are variables and constants in algebra?
In algebra, variables are symbols that represent unknown or changing quantities, typically denoted by letters like x, y, or z. They are used to formulate equations and expressions where the specific value is not yet known. On the other hand, constants are fixed values that do not change, such as numbers like 3, -7, or π. Constants remain the same throughout a mathematical operation or equation and do not vary.
What is the difference between equations and inequalities?
Equations are mathematical statements asserting that two expressions are equal, while inequalities are statements asserting a relationship between two expressions that are not necessarily equal. In equations, both sides are balanced and must be the same, whereas in inequalities, there is a comparison of values, such as greater than, less than, greater than or equal to, or less than or equal to. Equations have an equal sign, whereas inequalities have symbols like <, >, ≤, or ≥.
How do you solve multi-step equations?
To solve multi-step equations, start by simplifying each side of the equation by combining like terms. Then, isolate the variable by performing the inverse operation on both sides of the equation, working from the outermost terms to the innermost terms. Continue simplifying until you have the variable isolated on one side and the solution on the other side. Finally, check your solution by substituting it back into the original equation to ensure it satisfies the equation.
What is the difference between like terms and unlike terms?
Like terms are terms that have the same variables with the exact same exponent, making them comparable and combinable in algebraic expressions. On the other hand, unlike terms are terms that have different variables or the same variables with different exponents, meaning they cannot be combined directly.
How do you solve equations with variables on both sides?
To solve equations with variables on both sides, you first simplify the equation by combining like terms on each side to get a single variable term on each side. Then, move all the variable terms to one side of the equation by performing inverse operations (addition or subtraction) to isolate the variable on that side. Finally, solve for the variable by performing any necessary operations to get the variable by itself, and then calculate its value.
What are the different ways to represent a linear equation?
A linear equation can be represented in different ways, such as in slope-intercept form (y = mx + b), standard form (ax + by = c), point-slope form (y - y₁ = m(x - x₁)), and using two-point form (y - y₁ = (y₂ - y₁)/(x₂ - x₁) * (x - x₁)). Each form provides a unique way to describe the relationship between variables in a linear equation.
How do you solve word problems using algebraic expressions?
To solve word problems using algebraic expressions, you first need to identify the unknown quantity or variable in the problem, then create an algebraic expression that represents the relationship between the known and unknown quantities. Next, you can set up an equation based on the given information in the problem and solve for the unknown variable by simplifying and isolating it. Finally, you can check your solution by plugging it back into the original problem to ensure it satisfies all the conditions stated.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.






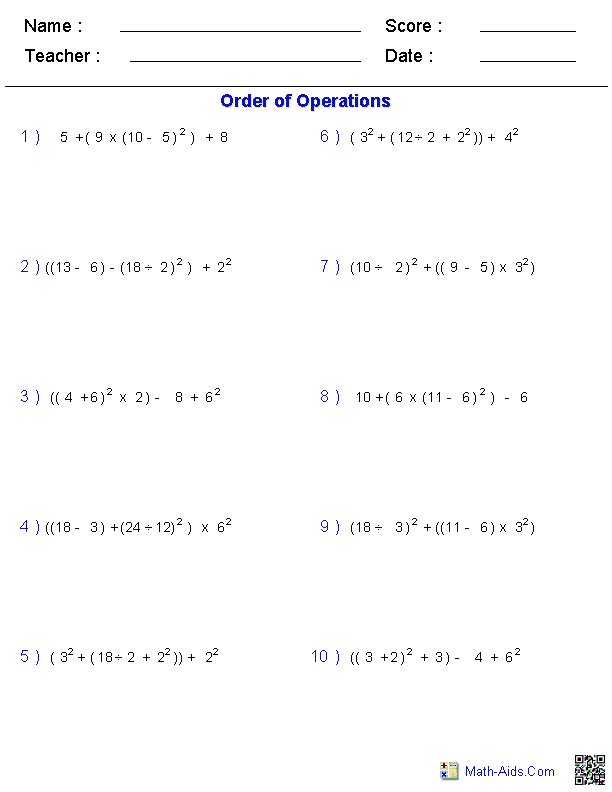
















Comments