3rd Grade Fraction Practice Worksheets
Are you a 3rd-grade teacher on the hunt for engaging and effective fraction practice worksheets? Look no further! Providing students with well-designed worksheets is a crucial element in enhancing their understanding of fractions. These practice worksheets serve as a valuable resource to reinforce concepts and develop their skills in a structured and engaging manner. With various topics and exercises tailored to the 3rd-grade curriculum, these worksheets will help your students grasp fractions with confidence and enthusiasm.
Table of Images 👆
- Fraction Practice Worksheets
- Printable 3rd Grade Math Worksheets Fractions
- 3rd Grade Math Worksheets Printable
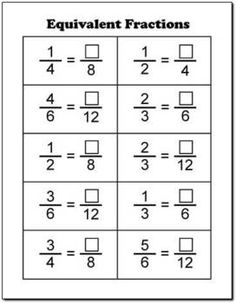
- Equivalent Fractions Worksheet 5th Grade
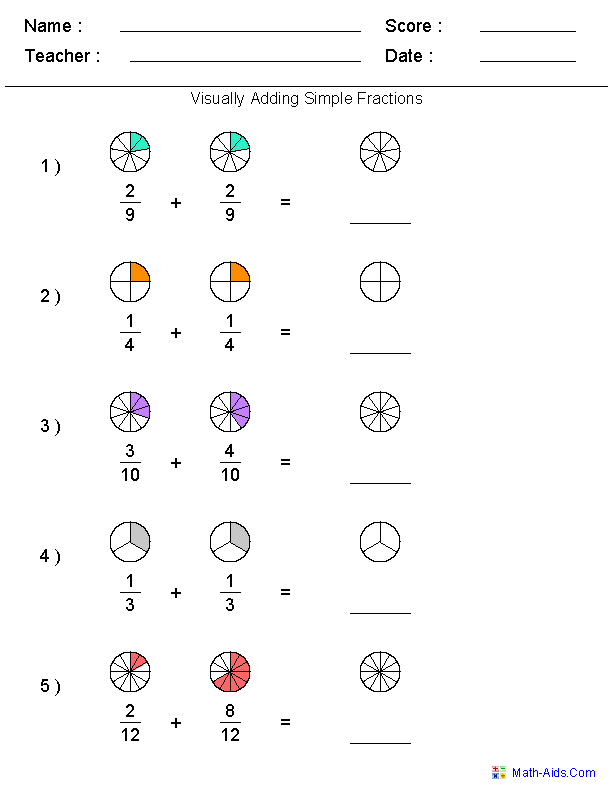
- Adding Fractions Worksheets Grade 4
- Fraction Practice Worksheets
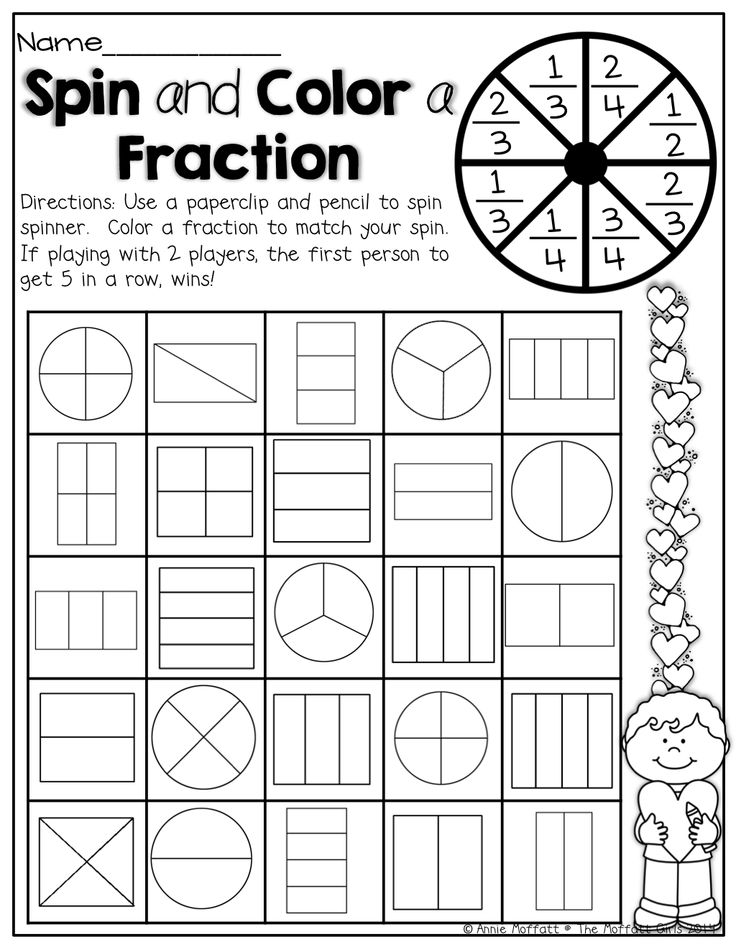
- First Grade Fraction Color
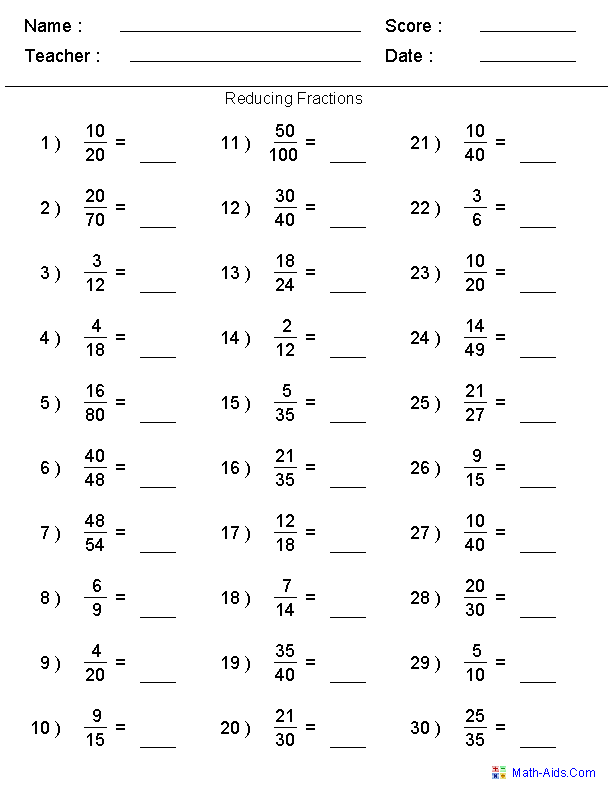
- Reducing Fractions Worksheet 5th Grade
- 6th Grade Math Worksheets Fractions
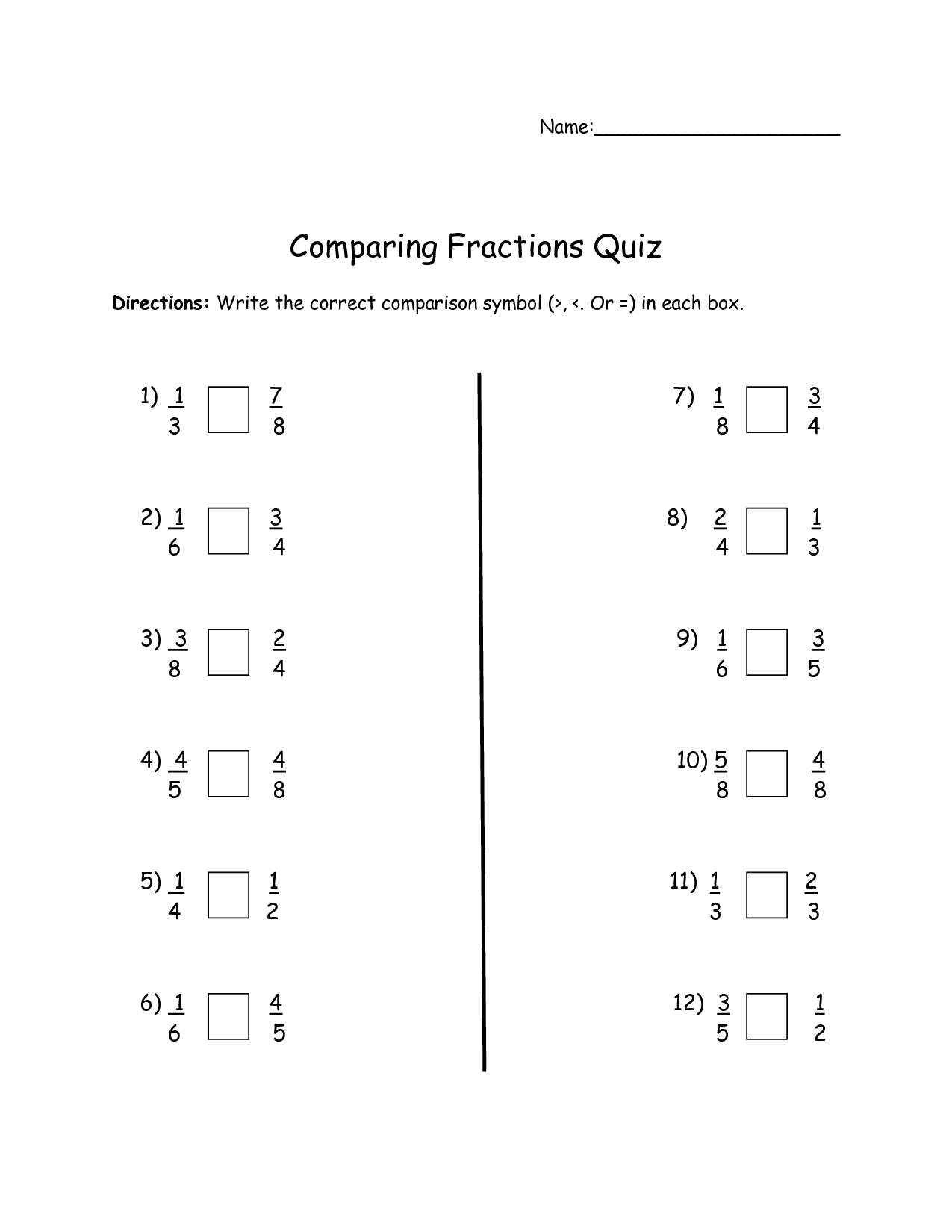
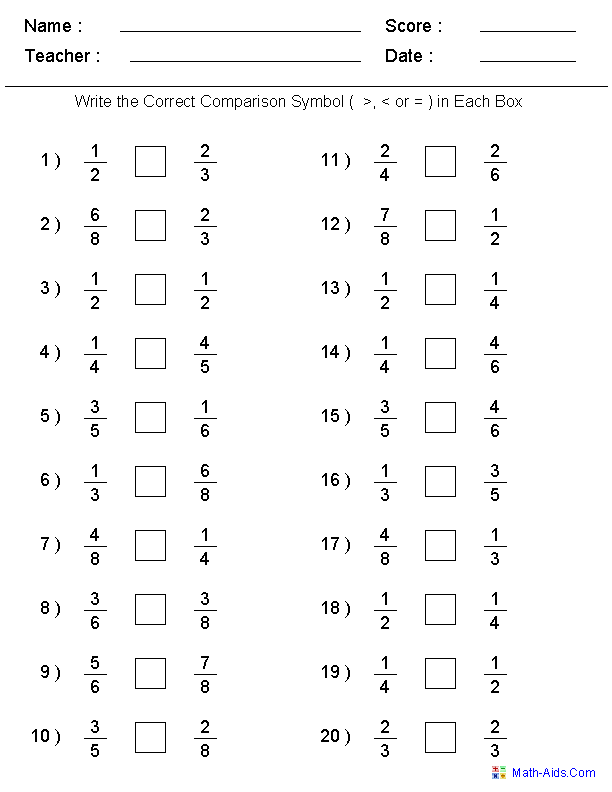
- Comparing Fractions Worksheets 3rd Grade
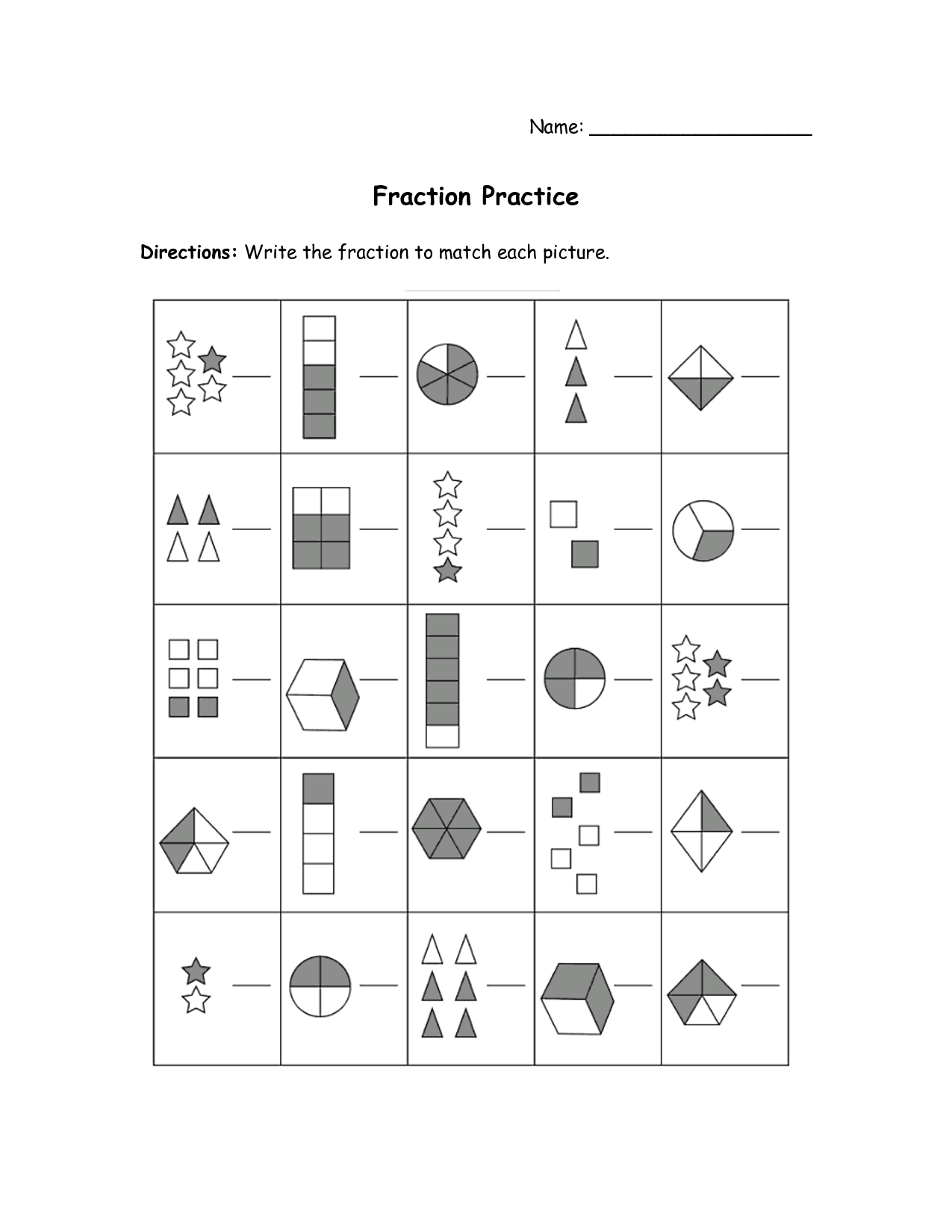
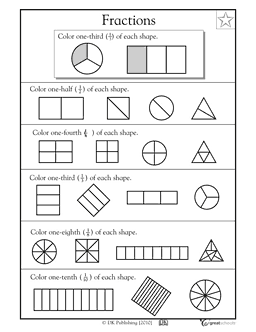
- Shape Fractions Worksheets Grade 1
- 3rd Grade Math Worksheets Geometry
- Comparing Fractions Worksheets 4th Grade
- 3rd Grade Math Worksheets Decimals
- Ordinal Numbers Worksheet Grade 1
- Equivalent Fractions Homework
More 3rd Grade Worksheets
Telling Time Worksheets 3rd GradeTime Worksheets for 3rd Grade
3rd Grade Reading Comprehension Worksheets
Energy Worksheets 3rd Grade Science
Multiplication Worksheets for 3rd Grade
3rd Grade Math Division Worksheets Printable
Short Reading Comprehension Worksheets 3rd Grade
Soil Worksheets for 3rd Grade
Cursive Writing Worksheets for 3rd Grade
3rd Grade Multiplication Properties Worksheet
What is a fraction?
A fraction is a numerical quantity that represents a part of a whole, expressed as one number divided by another with a horizontal line in between, for example, 1/2. It consists of a numerator (the top number) that represents the part being considered and a denominator (the bottom number) that represents the total number of parts that make up a whole.
How can you represent a fraction using a visual model?
One way to represent a fraction using a visual model is to use a bar or rectangular model. The bar or rectangle can be divided into equal parts to represent the denominator of the fraction. Then, the numerator can be represented by shading in the corresponding number of those parts to show the fraction visually. This helps to illustrate the relationship between the numerator and denominator in a clear and tangible way.
How do you compare two fractions?
To compare two fractions, you can either find a common denominator and compare the numerators, or convert both fractions to decimals and compare the values. By finding a common denominator, you can easily see which fraction is larger by comparing the numerators. Alternatively, converting both fractions to decimals will give you a numerical value that can be compared straightforwardly to determine which fraction is greater.
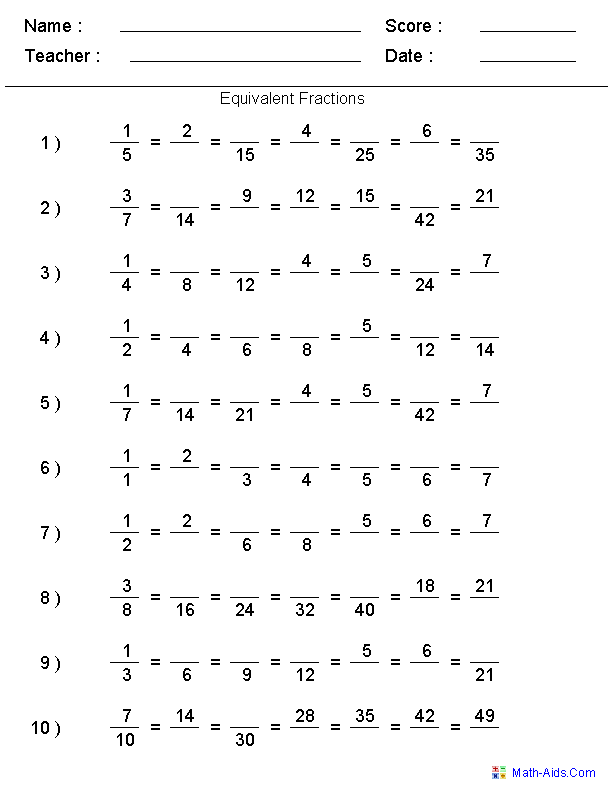
What is an equivalent fraction?
An equivalent fraction is a fraction that represents the same proportional amount as another fraction, but with different numerator and denominator values. This means that even though the numbers are different, the overall value or amount represented by the fractions is the same.
How do you simplify or reduce a fraction?
To simplify or reduce a fraction, divide the numerator and the denominator by their greatest common factor (GCF). This involves finding the largest number that both the numerator and denominator can be divided evenly by. Keep dividing until the numerator and denominator have no common factors other than 1. This will give you the simplest form of the fraction.
How do you add or subtract fractions with the same denominator?
To add or subtract fractions with the same denominator, you simply add or subtract the numerators and keep the denominator the same. For example, if you have 3/8 + 2/8, you would add the numerators to get 5 and keep the denominator as 8, resulting in 5/8. Similarly, if you have 5/6 - 2/6, you would subtract the numerators to get 3 and keep the denominator as 6, resulting in 3/6, which can be simplified to 1/2.
How do you add or subtract fractions with different denominators?
To add or subtract fractions with different denominators, you first need to find a common denominator. To do this, you can either find the least common multiple of the denominators or multiply the denominators of each fraction together. Once you have a common denominator, you can add or subtract the fractions as usual, keeping the denominator the same and adding or subtracting the numerators. Finally, simplify the resulting fraction if necessary by reducing it to its simplest form by dividing the numerator and denominator by their greatest common divisor.
How do you multiply a fraction by a whole number?
To multiply a fraction by a whole number, you simply multiply the numerator of the fraction by the whole number, and leave the denominator unchanged. For example, to multiply 2/3 by 4, you would multiply the numerator (2) by 4 to get 8, and keep the denominator (3) the same, resulting in 8/3.
How do you multiply two fractions?
To multiply two fractions, you simply multiply the numerators (top numbers) together to get the new numerator, and then multiply the denominators (bottom numbers) together to get the new denominator. This gives you the product in the form of a fraction. Remember to simplify the fraction if possible by reducing it to its simplest form by dividing both the numerator and denominator by their greatest common factor.
How do you divide a fraction by a whole number?
To divide a fraction by a whole number, you can convert the whole number into a fraction by putting it over 1, then multiply the fraction by the reciprocal of the fraction representing the whole number. This means flipping the whole number fraction upside down and multiplying it with the original fraction to get the final quotient. Remember to simplify the resulting fraction if possible.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.

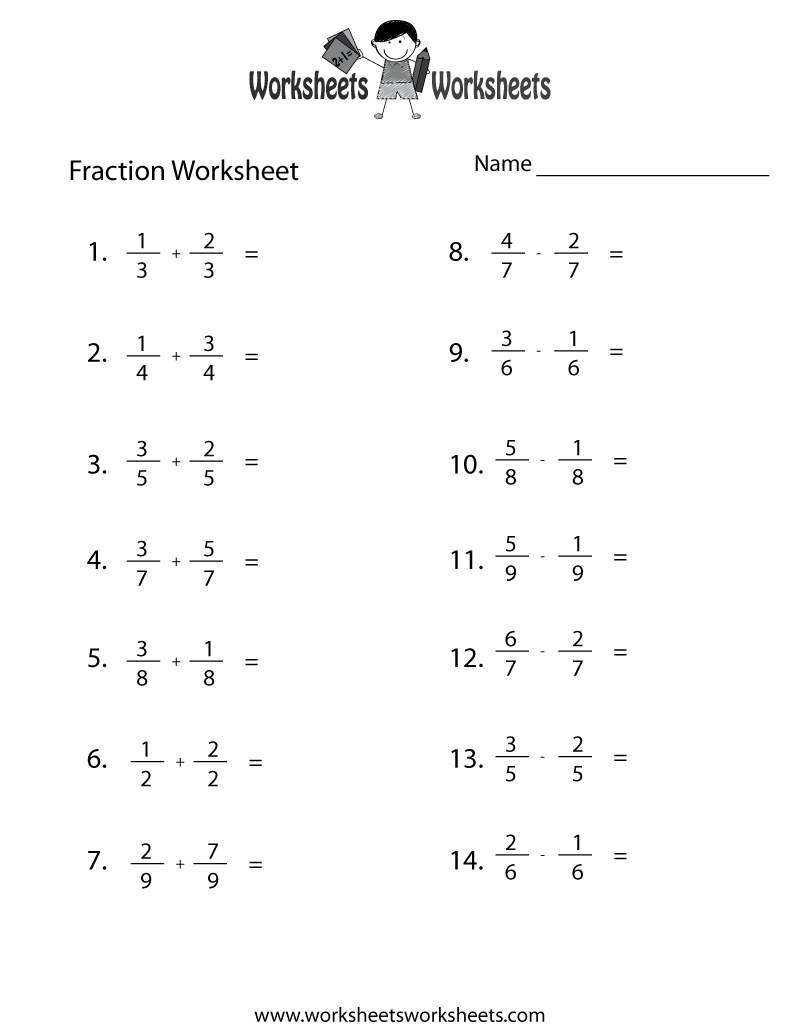



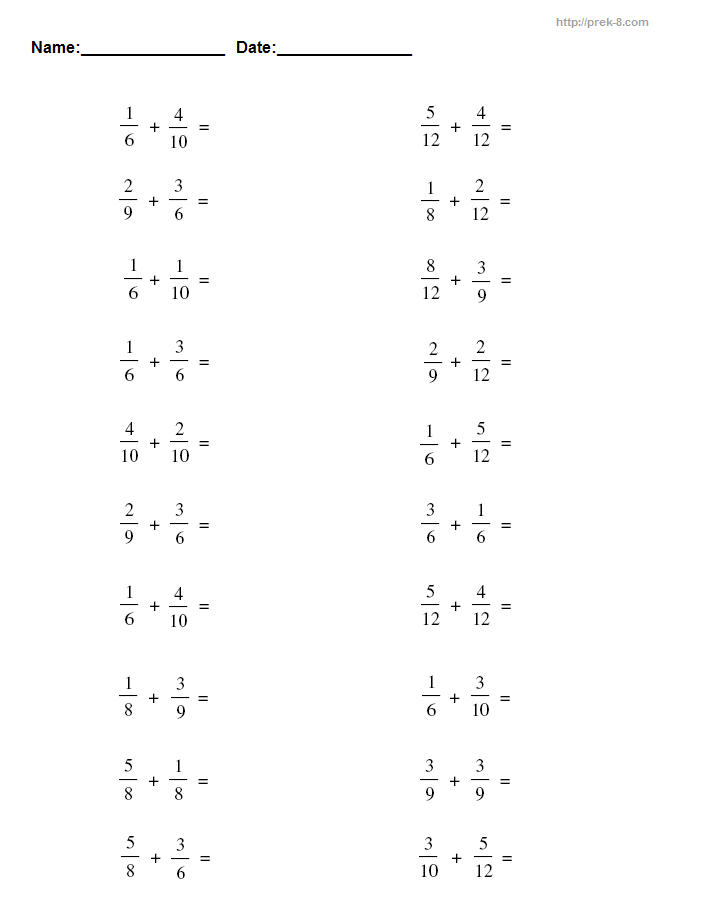
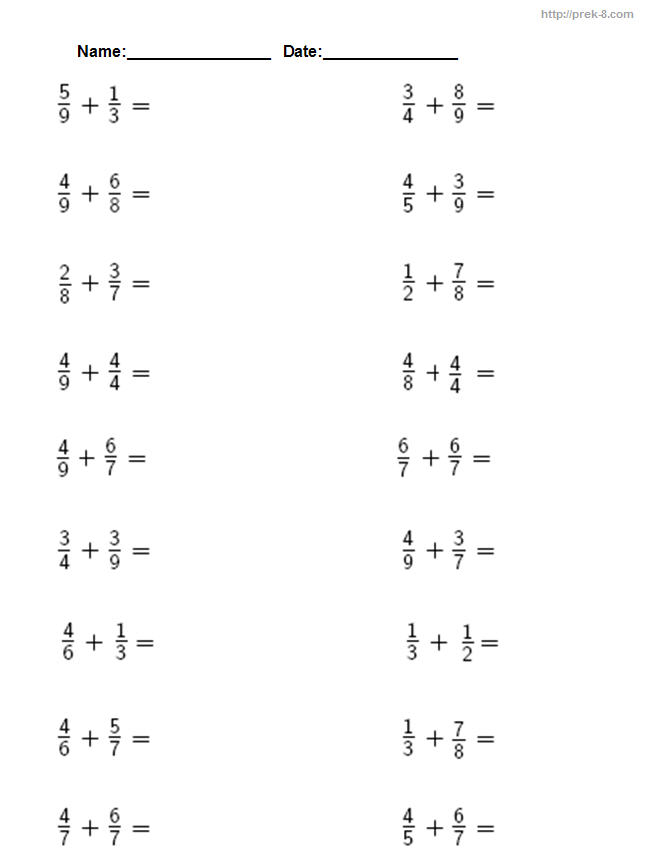
















Comments