12th Grade Math Worksheets
Are you a 12th grade student searching for effective study resources to enhance your math skills? Look no further! We have a wide range of math worksheets specifically designed for students at your academic level. These worksheets cater to various mathematical concepts and topics, making them an ideal supplement for your classroom learning. With our comprehensive collection of 12th grade math worksheets, you can confidently tackle complex equations and problems while reinforcing your understanding of key mathematical principles.
Table of Images 👆
More Math Worksheets
Printable Math WorksheetsMath Worksheets Printable
Printable Math Worksheets Multiplication
Math Worksheets for 2nd Graders
Math Practice Worksheet Grade 6
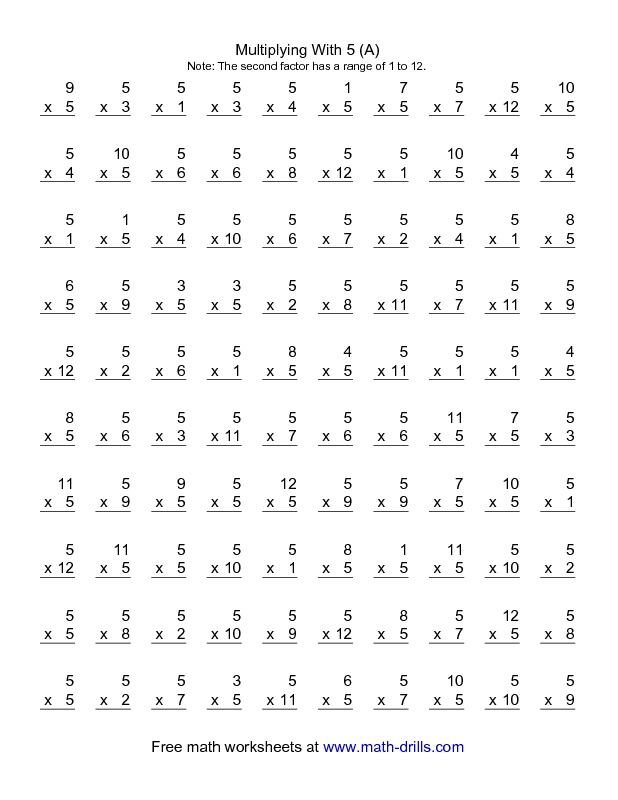
Math Multiplication Worksheets
First Grade Subtraction Math Worksheets Printable
Rocket Math Practice Worksheets
Math Worksheets Integers
Superhero Math Worksheets
What is a quadratic equation?
A quadratic equation is a second-degree polynomial equation that can be written in the form ax^2 + bx + c = 0, where x represents an unknown variable, and a, b, and c are constants with a not equal to zero. Quadratic equations always have a highest power of x squared, and they typically have two solutions known as roots. These equations are commonly used in algebra and can be solved using various methods such as factoring, completing the square, or using the quadratic formula.
How do you find the derivative of a function?
To find the derivative of a function, you need to apply the rules of differentiation, such as the power rule, product rule, chain rule, or quotient rule depending on the function's form. You differentiate each term of the function with respect to the independent variable, typically denoted as x, and simplify the expression to find the derivative. Remember to practice and familiarize yourself with the different rules and techniques to efficiently find derivatives.
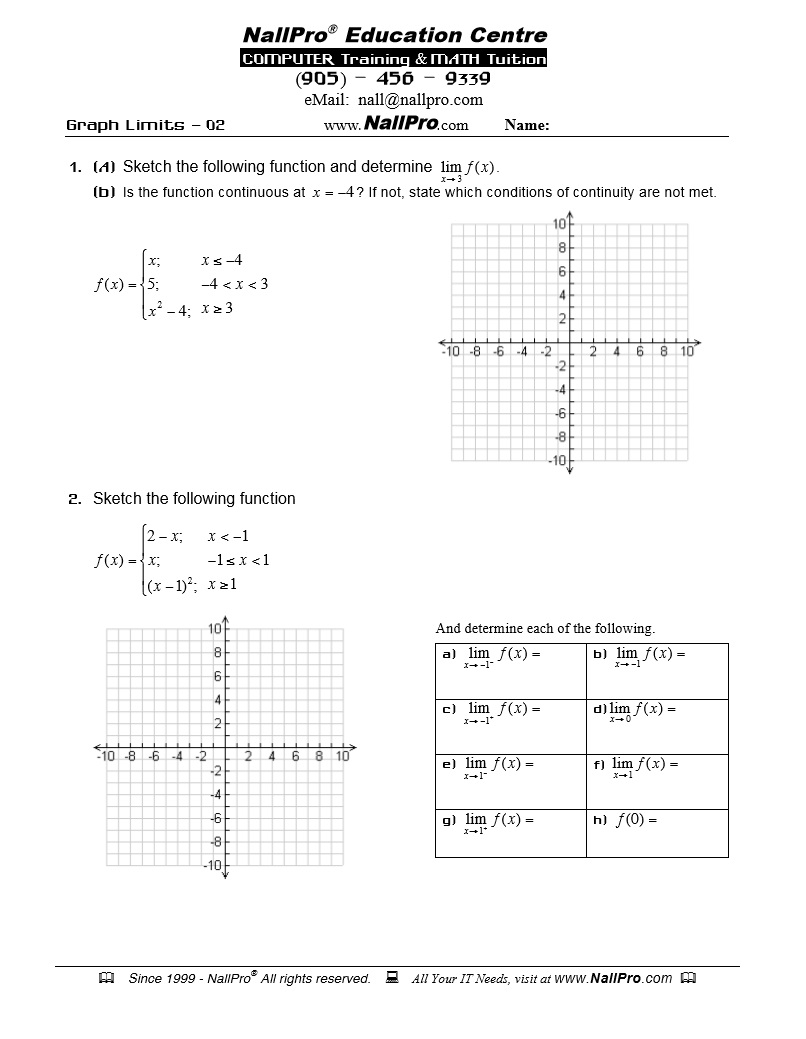
What is the concept of limits in calculus?
In calculus, the concept of limits is used to describe the behavior of a function as the input approaches a certain value. It involves understanding how a function behaves as it gets closer and closer to a particular point or value, without necessarily reaching it. Limits are fundamental to calculus as they help in defining derivatives, integrals, and continuity of functions, providing a way to study the behavior of functions in a precise and rigorous manner.
How do you solve trigonometric equations?
To solve trigonometric equations, you typically use trigonometric identities or properties to simplify the equation, reduce it to a known form, and then solve for the unknown variable. You may also use the unit circle, periodic properties of trigonometric functions, and reciprocal relationships to manipulate the equations. Additionally, factoring and applying algebraic techniques may be necessary in some cases. It is important to be familiar with trigonometric functions, identities, and properties to effectively solve trigonometric equations.
How do you calculate the probability of an event?
To calculate the probability of an event, you divide the number of favorable outcomes by the total number of possible outcomes. The resulting fraction or decimal represents the likelihood of that event occurring. Probability is typically expressed as a value between 0 and 1, where 0 means the event is impossible and 1 means the event is certain.
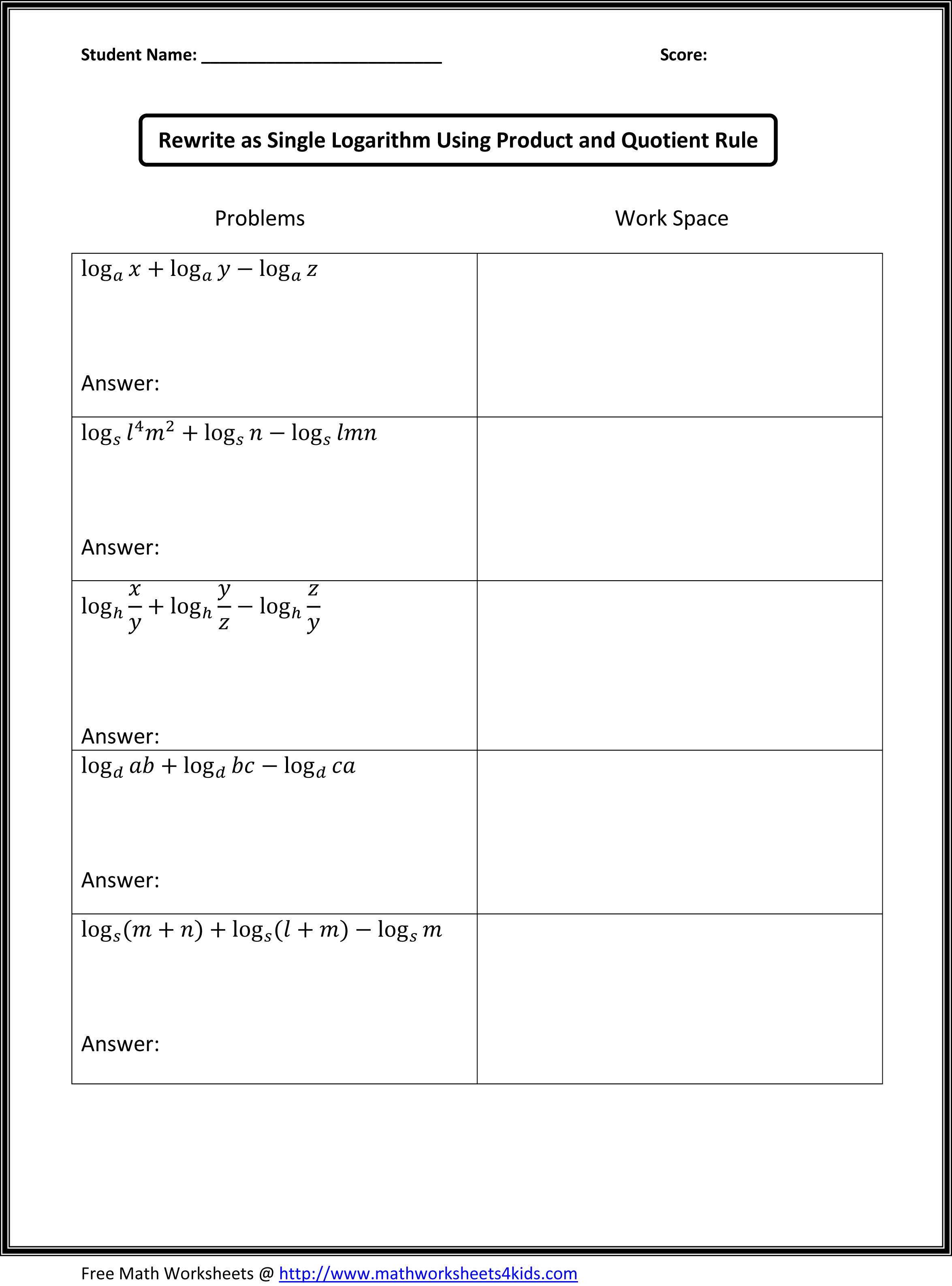
What are the properties of logarithmic functions?
Logarithmic functions are inverses of exponential functions, represented as \( f(x) = \log_b(x) \). Key properties include the domain as \( x > 0 \), vertical asymptote at \( x = 0 \), x-intercept at \( x = 1 \), and range as all real numbers. Logarithmic functions also exhibit the properties of shifting vertically and horizontally, as well as stretching or compressing their graphs. Additionally, the logarithmic function \( f(x) = \log_b(x) \) can be rewritten in exponential form as the base \( b \) raised to the power of \( f(x) \) equals \( x \), offering a bijective relationship.
How do you find the area of irregular shapes using integration?
To find the area of an irregular shape using integration, you can break down the shape into simpler geometric figures or curves, then express their boundaries through functions in terms of one variable. By integrating these functions within the specified bounds, you can calculate the area enclosed by the irregular shape. This method is particularly useful for shapes that cannot be easily measured or computed using traditional geometric formulas.
What is the difference between permutations and combinations?
Permutations are arrangements of objects where the order matters, while combinations are selections of objects where the order does not matter. In permutations, the order of the elements matters, whereas in combinations, the order does not affect the result.
How do you solve systems of linear equations using matrices?
To solve systems of linear equations using matrices, you first write the system of equations in matrix form, where the coefficients of the variables are placed in a matrix called the coefficient matrix. Then, you create a matrix augmented with the constants on the right side of the equations. Next, you perform row operations to transform the augmented matrix into reduced row-echelon form by applying operations like row addition, multiplication, and swapping. Once in this form, the solutions can be easily read off the matrix. If the matrix is in inconsistent form, this means the system has no solution. If the matrix is in consistent form, the values of the variables can be extracted to solve the system of linear equations.
What are the properties of conic sections, such as circles and ellipses?
Conic sections, including circles and ellipses, have common properties such as being formed by the intersection of a plane with a double cone. Circles are a special case of ellipses with constant distance from the center to any point on the circumference, while ellipses have two focal points where the sum of the distances to any point on the curve remains constant. Both shapes exhibit symmetry, have major and minor axes, and can be described by their eccentricity, which determines their elongation. Circles have an eccentricity of zero, making them perfectly round, while ellipses have an eccentricity between 0 and 1, dictating their degree of elongation.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.
























Comments