12th Grade Math Worksheets Problems

12th grade math is known for its complex problem-solving and critical thinking skills. To reinforce these skills, having access to suitable worksheets can be incredibly helpful. Whether you're a student or a teacher looking for supplementary resources, worksheets provide an effective way to practice and assess understanding. With a range of topics and a focus on various mathematical concepts, these worksheets can play a vital role in enhancing your mathematical abilities.

  Printable Math Addition Worksheets

---

  4th Grade Math Homework Worksheets

---

  12 Grade Math Worksheets

---

  2nd Grade Math Worksheets Multiplication

---

  7th Grade Math Worksheets

---

  8th Grade Math Problems Worksheets

---

What is the value of x in the equation 3x + 8 = 20?

The value of x in the equation 3x + 8 = 20 is x = 4.

Find the derivative of y = 2x^3 - 5x^2 + 7x - 4.

The derivative of y = 2x^3 - 5x^2 + 7x - 4 is given by dy/dx = 6x^2 - 10x + 7.

Solve the system of equations: 2x + 3y = 10 and 4x - y = 6.

To solve the system of equations, first solve for y in the second equation by adding y to both sides to get y = 4x - 6. Substituting this into the first equation, we get 2x + 3(4x - 6) = 10. Simplifying this gives 14x - 18 = 10. Add 18 to both sides to get 14x = 28, then divide by 14 to find x = 2. Substituting x back into y = 4x - 6 from before gives y = 4(2) - 6, so y = 2. Therefore, the solution to the system of equations is x = 2 and y = 2.

Evaluate the integral ∫ (4x^2 + 3x + 1) dx.

The integral of (4x^2 + 3x + 1) dx is equal to (4/3)x^3 + (3/2)x^2 + x + C, where C is the constant of integration.

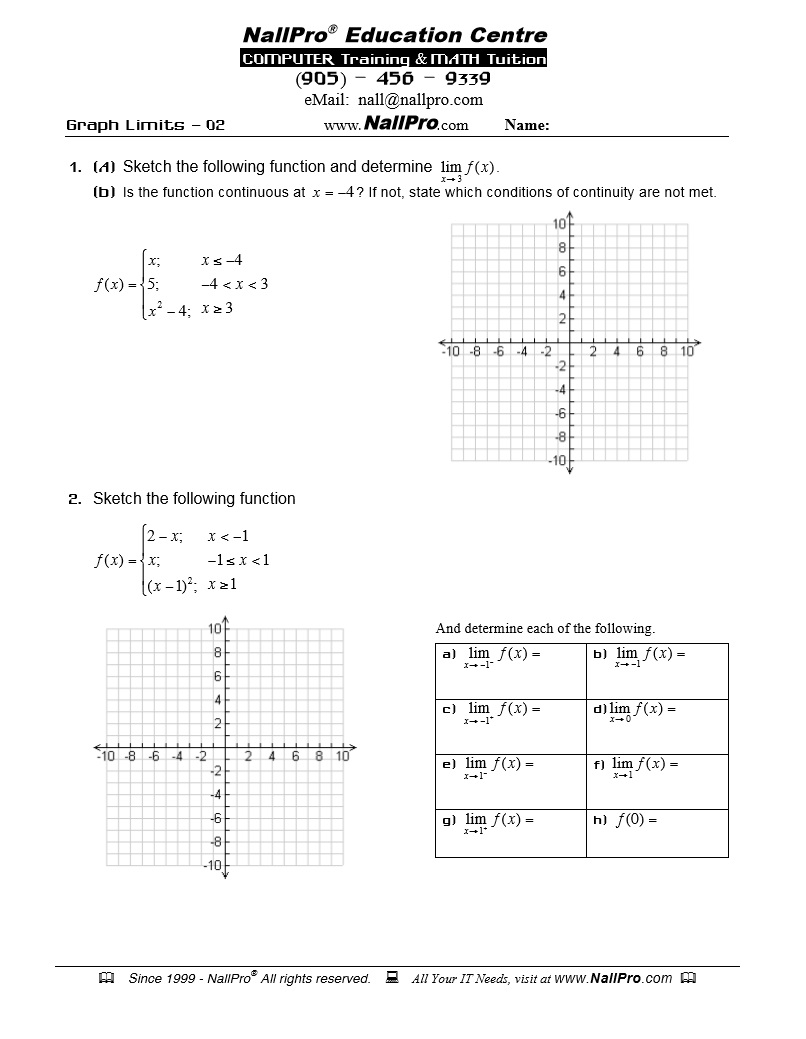
Determine the domain of the function f(x) = √(9 - x^2).

The domain of the function f(x) = √(9 - x^2) is all real numbers such that -3 ≤ x ≤ 3. This is because the square root function is defined only for non-negative values, and the expression inside the square root must be greater than or equal to 0. Thus, for f(x) to be defined, 9 - x^2 ≥ 0, which gives the domain as -3 ≤ x ≤ 3.

Find the vertex of the parabola given by the equation y = -2x^2 + 8x - 7.

The vertex of the parabola given by the equation y = -2x^2 + 8x - 7 is found by calculating the x-coordinate using the formula x = -b/(2a), where a = -2 and b = 8. Substituting these values in, we get x = -8 / (2*(-2)) = 2. To find the corresponding y-coordinate, substitute x = 2 back into the equation y = -2x^2 + 8x - 7 to get y = -2(2)^2 + 8(2) - 7 = -8 + 16 - 7 = 1. Therefore, the vertex of the parabola is (2, 1).

Calculate the probability of rolling two dice and getting a sum of 7.

The sum of 7 can be made in 6 ways out of a total of 36 possible outcomes when rolling two dice (1+6, 2+5, 3+4, 4+3, 5+2, 6+1). Therefore, the probability of rolling two dice and getting a sum of 7 is 6/36, which simplifies to 1/6 or approximately 16.67%.

Evaluate the limit lim(x→∞) (3x - 5) / (2x + 1).

To evaluate the limit lim(x→∞) (3x - 5) / (2x + 1), we can simplify the expression by dividing every term by x. This gives us (3 - 5/x) / (2 + 1/x). As x approaches infinity, both 5/x and 1/x will approach 0. Therefore, the limit simplifies to (3 - 0) / (2 + 0), which equals 3/2. So, the limit of the given expression as x approaches infinity is 3/2.

Find the equation of the line that passes through the points (2, 4) and (6, -1).

To find the equation of the line passing through the points (2, 4) and (6, -1), first find the slope using the formula (y2 - y1) / (x2 - x1). Substituting the coordinates, the slope is (-1 - 4) / (6 - 2) = -5 / 4. Next, use the point-slope form of a line, y - y1 = m(x - x1), where m is the slope and (x1, y1) are the coordinates of one of the points given. Substituting (2, 4), the equation becomes y - 4 = (-5/4)(x - 2). Simplifying gives the equation of the line as y = -5x/4 + 13/2.

Determine the inverse function of f(x) = 2x - 3.

To find the inverse function of f(x) = 2x - 3, first, switch the roles of x and y: x = 2y - 3. Next, solve for y: (x + 3)/2 = y. Therefore, the inverse function of f(x) = 2x - 3 is f^(-1)(x) = (x + 3)/2.