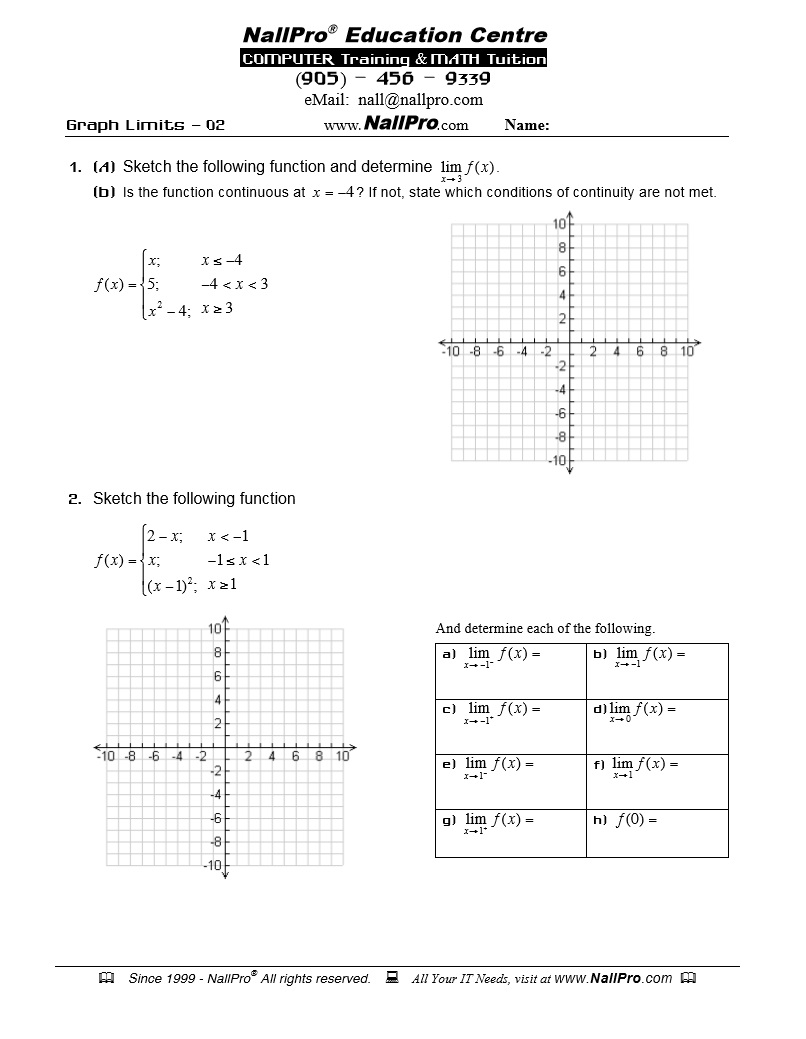
12th Grade Algebra Worksheets
For high school seniors who are seeking additional practice and review in algebra, 12th Grade Algebra Worksheets offer a valuable resource. These worksheets provide a range of exercises and problems that cover important topics and concepts from the curriculum. With a focus on helping students strengthen their understanding and build confidence, these worksheets serve as a helpful tool for mastering algebraic concepts in preparation for college and beyond.
Table of Images 👆
More Other Worksheets
Kindergarten Worksheet My RoomSpanish Verb Worksheets
Cooking Vocabulary Worksheet
My Shadow Worksheet
Large Printable Blank Pyramid Worksheet
Relationship Circles Worksheet
DNA Code Worksheet
Meiosis Worksheet Answer Key
Art Handouts and Worksheets
7 Elements of Art Worksheets
What are some applications of algebra in real life?
Algebra is widely used in real life applications such as budgeting and financial planning, calculating measurements and proportions in construction and engineering, analyzing data in scientific research, designing and creating computer graphics in technology, and even predicting trends and patterns in business and economics. Overall, algebra helps in problem-solving, decision-making, and understanding the relationships between different variables in various fields.
How can we solve linear equations with one variable?
To solve linear equations with one variable, you first need to isolate the variable on one side of the equation by using inverse operations. Start by simplifying both sides of the equation if needed, then perform addition, subtraction, multiplication, or division to get the variable alone on one side. Finally, check your solution by plugging it back into the original equation to ensure it satisfies the equation.
What are the different methods for solving quadratic equations?
The different methods for solving quadratic equations include factoring, using the quadratic formula, completing the square, and graphing. Each method has its own advantages and can be chosen based on the context of the equation and the ease of solving it.
How can we simplify expressions using exponents?
To simplify expressions using exponents, you can apply the rules of exponents, such as multiplying exponents when the bases are the same, dividing exponents when dividing like bases, and raising a power to a power by multiplying the exponents. Combine like terms and simplify the resulting expression by following the rules of exponents to achieve a simplified form. Remember to also consider negative exponents and the zero exponent rule for cases where the exponent is zero.
How do we graph linear functions?
To graph a linear function, first obtain the equation in the form y = mx + b where m is the slope and b is the y-intercept. Plot the y-intercept on the y-axis as a point (0, b). Then, use the slope to find another point on the line by moving up or down based on the rise and left or right based on the run. Connect the two points with a straight line to represent the linear function on the graph. Repeat this process if needed for additional points to ensure accuracy and to extend the line as necessary.
What are the properties of logarithms?
Logarithms have several key properties, including the product rule (log(xy) = log(x) + log(y)), the quotient rule (log(x/y) = log(x) - log(y)), the power rule (log(x^n) = n*log(x)), the change of base formula (log_b(x) = log_c(x) / log_c(b)), and the inverse properties (log_b(b^x) = x and b^(log_b(x)) = x). Additionally, the logarithms of 1 and 0 are equal to 0 and undefined, respectively.
How can we solve systems of equations using substitution?
To solve systems of equations using substitution, first isolate one variable in one of the equations. Then, substitute that expression into the other equations, replacing the variable with the expression. This will result in a new equation with only one variable, which can be solved to find its value. Substituting this value back into one of the original equations will help you solve for the other variable. Repeat this process until you have found values for all variables, giving you the solution to the system of equations.
What are the steps for factoring polynomials?
To factor a polynomial, you first look for any common factors among all the terms and factor them out. Then, you can try different methods such as grouping, difference of squares, or using special factoring formulas to factor the polynomial further. Finally, you ensure that the factored form is in its simplest form and cannot be factored any further.
How do we find the domain and range of a function?
To find the domain of a function, determine all possible input values that the function can accept. Look for any restrictions such as division by zero or square roots of negative numbers. To find the range, identify all the output values that the function can produce. Explore the behavior of the function as the input changes to understand the range of possible output values. Graphical representations and algebraic techniques can also help in finding the domain and range of a function.
How can we solve word problems involving algebraic equations?
To solve word problems involving algebraic equations, first identify the unknown variable and represent it with a letter. Translate the problem into an equation using the given information and the relationships described in the problem. Solve the equation by performing the necessary operations to isolate the variable. Finally, check your solution by plugging it back into the original equation to ensure it satisfies the conditions in the problem. Practice and understanding of algebraic concepts will enhance proficiency in solving word problems involving algebraic equations.
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.

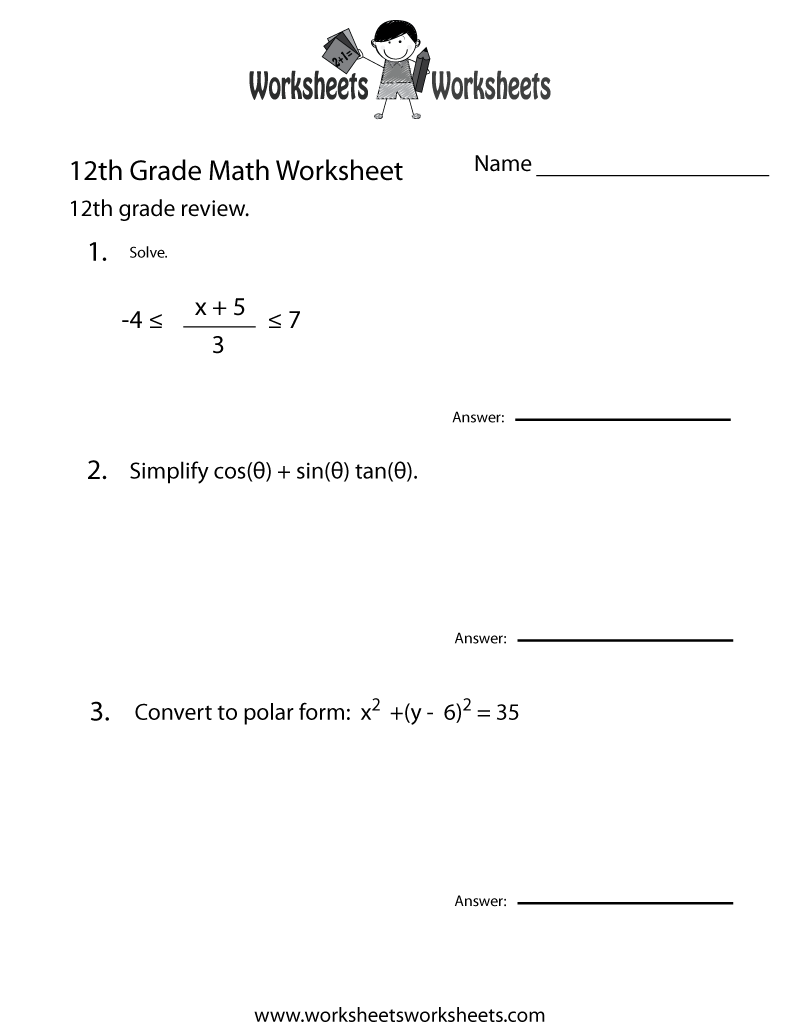
























Comments